Introduction

Nanostructured lipid carrier (NLC) is an updated version of solid lipid (SL) nanoparticles (SLN). SLN was modified with the purpose to enhance loading capacity (LC) while decreasing time-dependent drug expulsion. This enhanced functionality of NLC was due to the incorporation of liquid lipid (LL) in the SL matrix which disorganizes SL providing more space for the drug to accommodate and remain there without expulsion [1, 2]. NLC is widely used in the administration of drugs through oral, intranasal, ocular, topical, and parenteral routes without compromise in its stability [3–7]. NLC can also enhance the bioavailability of a wide range of therapeutics [8, 9]. NLC is made of components that are generally recognized as safe by Food and Drug Administration (FDA) [9]. Furthermore, NLC also acts as a controlled and site-specific drug delivery system [8, 10, 11].

The application of quality by design (QbD) in the development of NLC has sharply increased during the last decades because of the realization that quality cannot be tested into the final product but should be built in by design [12, 13]. Conventionally, the development of pharmaceutical products and the assurance of their quality were based on controlling raw materials and processes used. If a product does not fulfill the regulatory specifications, then the pharmaceutical company has to restart the manufacturing process again to identify the cause of out of specifications. Such a procedure of testing incurred more costs to the company and also decreases the safety of the final dosage form. To avoid these limitations, the concept of QbD was adapted, which not only minimizes batch failure thus saving company costs but also ensures the quality and safety of the finished dosage form. Several benefits of QbD in the development of a pharmaceutical product is represented in Figure 1 [14, 15].

Display full size

Figure 1. 

Advantages of QbD over the conventional approach

Adaptation of QbD in the development of lipid-based nanocarriers is a new approach but the term was introduced in 1992 by Juran [16]. However, Juran [16] did not use the terms drug, excipients, and medical devices in his book. Due to quality concerns in the pharmaceutical product United States FDA in 2004 integrated a quality system and risk-based approach [17]. A few years later International Conference on Harmonization (ICH) introduced the term QbD in ICH Q8 which is officially defined as a systematic approach to development that begins with predefined objectives and emphasizes product and process understanding and process control, based on sound science and quality risk management (QRM) [18]. ICH Q9 guideline is based on the risk management principle to identify and control the variables significantly affecting the quality of the product [19]. ICH Q10 gives guidance on pharmaceutical quality system and includes good manufacturing practice (GMP) regulations and complements ICH Q8 and ICH Q9 [20]. It can be said that if ICH Q8, Q9, and Q10 guidelines are implemented can deliver robust pharmaceutical products with consistent quality [21].

The application of these guidelines in pharmaceutical development is complex and time-consuming as there are so many independent variables (inputs) that can significantly affect the dependent variables (output or response). Studying the effect of each combination to understand the relationship between input and output is not easy to understand. Consequently, to explore the relationship effectively a statistical tool called the design of experiment (DoE) is utilized which is a structured approach to the development of a product within the QbD framework [22, 23].

Earlier to DoE one factor at a time (OFAT) approach was used. This method involves studying the effect on dependent variables by varying OFAT and keeping other factors constant until no significant effect of one or more variables on response is obtained. This method is complex, time-consuming, and also increases the cost to the company. Furthermore, if two or more factors are varied simultaneously then the effect of the interaction of these variables on response cannot be identified [23–25].

Integration of the concept of six sigma finds out ways to improve and modify the process, which yields nanoparticles that have no quality defect and give more customer satisfaction. This concept has not been implemented in the industry so far for the formulation of lipid-based nanoparticles. Six sigma concepts have been described briefly in a future perspective.

This review aims at the application of QbD in the development of NLC. In doing so, firstly different elements of QbD are discussed followed by the discussion of the implementation of DoE in the systematic development of NLC within the design space.

Elements of QbD

Below figure indicates various stages in product development (Figure 2). It shows how different elements of QbD are interlinked with each other in the final production of NLC within the design space. Each of the elements is further described below.

Display full size

Figure 2. 

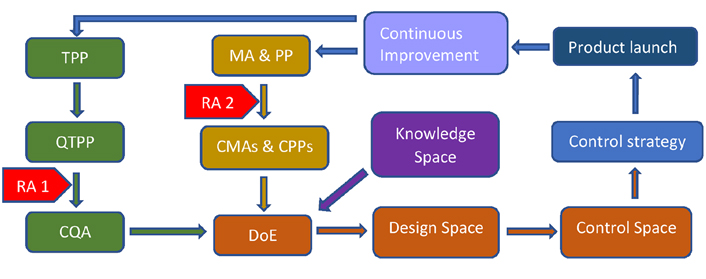
Life-cycle in the development of the pharmaceutical product by QbD implementation. From the target product profile (TPP), quality TPP (QTPP) is chosen and then risk assessment 1 (RA 1) is applied to analyze, which QTPP can be critical quality attributes (CQAs). RA 1 is nothing but just the analysis to select a particular QTPP as CQA. RA 2 refers to failure mode and effect analysis (FMEA) to select critical material attributes (CMAs) and critical process parameters (CPPs) from material attributes (MAs) and process parameters (PPs) respectively. CMAs, CPPs, and CQA are then fed into DoE software to get design space and control space. Further control of CMAs and CPPs is control strategy, which ensures batch-to-batch consistency of the product. TPP, MA, and PP should be updated if the necessity arises for further improvement of product quality

QRM

QRM consists of the identification of the severity of the risk and how to avoid or control it to the acceptable level so that it does not generate a quality failure. QRM is divided into 3 steps namely RA, risk control, and risk review [19].

RA

RA is the first step in QRM. It is further divided into risk identification, risk analysis, and risk evaluation. Risk can be assessed at two different steps as depicted in Figure 2. RA 1 is carried out to identify CQAs from QTPP while RA 2 is carried out to identify CMAs and CPPs from MAs and PPs respectively.

Risk identification

In order to identify whether any specific event or operation (MAs and/or PPs) is putting a risk on CQAs, the Ishikawa fishbone diagram was constructed (Figure 3). This diagram contains all the possible variables that can significantly affect CQAs. Each variable can affect CQAs to a different degree [30, 31].

Display full size

Figure 3. 

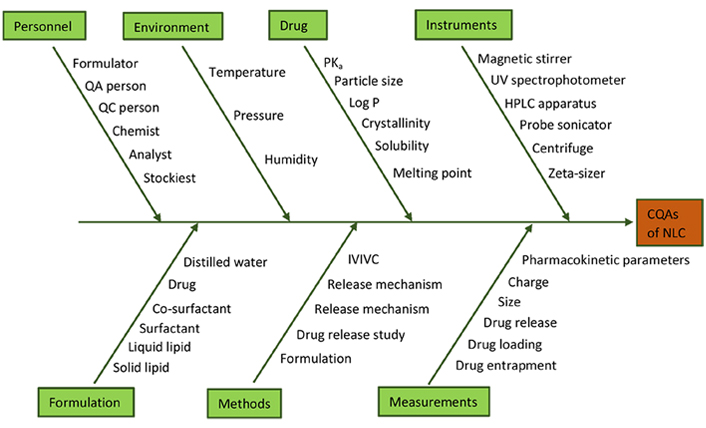
Ishikawa fish bone diagram illustrating the influence of different parameters on CQAs of NLC. HPLC: high-performance liquid chromatography; IVIVC: in vitro-in vivo correlation; Log P: partition coefficient; PK_a: acid dissociation constant; QA: quality assurance; QC: quality control; UV: ultraviolet-visible

Risk analysis and risk evaluation

To quantify how severe a factor can impact CQAs, risk priority number (RPN; RPN = severity × occurrence × detectability) for each factor is calculated. RPN measures whether MAs and PPs are at high, low, or moderate risk for the quality of the product. FMEA was performed to prioritize the risk factors based on RPN (Table 3). FMEA refers to the mode of failure and the factors responsible for such failures while effect analysis refers to studying the effect of those failures on product performance. Here product performance is related to CQAs [29]. It can be said that FMEA identifies CQA which is mainly responsible for causing product failure. Each parameter, i.e., severity, occurrence, and detectability are assigned scores from 1 to 5 which are substituted in the equation given below to calculate the RPN. Risk factors assigned medium and high RPN were further subjected to optimization studies.

Table 3.

FMEA matrix to identify the criticality of failure modes on the basis of RPN scores

S. No.	Failure modes	Severity (S)	Occurrence (O)	Detection (D)	RPN (S × O × D)	Risk
1	Stirring speed (rpm)	4	3	2	24	Medium
2	Stirring time (min)	4	4	2	32	Medium
3	SA (%)	4	5	2	40	Medium
4	ST (min)	5	5	2	50	High
5	Total lipid conc. (%)	5	5	3	75	High
6	Surfactant conc. (%)	5	5	3	75	High
7	Rate of mixing	2	1	2	4	Low
8	Temperature (°C)	3	3	2	18	Medium
9	Bead size	2	2	1	4	Low
10	Bead shape	1	1	1	1	Low
11	Beaker size	2	2	1	4	Low
12	Beaker color	1	1	1	1	Low

Display full size

conc.: concentration; rpm: rotation per minute; SA: sonication amplitude; ST: sonication time

Severity refers to how severe the impact of failure mode would be on the product quality. Severity is ranked as 5-catastrophic, 4-critical, 3-serious, 2-minor, and 1-no effect. Occurrence refers to how frequently a particular failure mode can affect product quality. Occurrence is ranked as 5-frequent, 4-probable, 3-occasional, 2-remote, and 1-unlikely. Detectability refers to the ease with which a failure mode can be detected to avoid product failure. Detectability is ranked as 5-hard to detect, 4-low or remotely detectable, 3-moderately detectable, 2-highly detectable, 1-easily detectable [30].

Risk control

Once the risk is identified and evaluated it is required to be reduced and/or accepted. Risk reduction is necessary to bring the quality of the product within an acceptable range [19]. High ST (risk) decreases drug EE (CQA) of NLC [32]. This results in an increase in the volume of this formulation to be taken by the patient provided the concentration of total lipids in the formulation is kept constant. If the route of administration is ocular (30 μL) or intranasal (200 μL) where the volume of the formulation required to be kept is minimum to avoid spillage then ST can result in product failure by decreasing the dose of the drug in the same volume of the formulation [33–35]. This product failure can be avoided by keeping a minimum ST during the formulation of NLC. Another example of risk minimization is found in the study research work of Lakhani and colleagues [36] who studied that 1,2-distearoyl-sn-glycero-3-phosphoethanolamine- N-[methoxy(polyethyleneglycol)] (mPEG-2K-DSPE) is essential for drug loading (DL) of 0.1% w/v of amphotericin B (AmB) but for 0.1% w/v of AmB, mPEG-2K-DSPE decreases DL. The possibility of DL of even 0.1% w/v diminishes if mPEG-2K-DSPE is omitted from formulation so mPEG-2K-DSPE being a risk is used at minimum concentration [36]. Sometimes there is a possibility that risk cannot be minimized so it has to be accepted. Risk acceptance is always done in that case when risk is not severely harming the patients, i.e., benefit to risk ratio is high. One example is the use of drugs (CMAs) in preparing NLC. Drugs particularly anticancer have several side-effects but they cannot be either omitted or used in less concentration in the formulation as it is the main active constituent for the treatment of cancer where the benefit to risk ratio is high.

Risk review

This is the final step of QRM where risks are reviewed or monitored which is based on prior experience and knowledge. Identification of CQAs (from QTPP) and recognition of CMAs and CPPs from MAs and PPs respectively requires prior knowledge and experience. Here prior knowledge refers to the formulation of NLC. Once the ingredients are selected, the formulation is prepared to further identify which excipient and process significantly impact the development of NLC. Various RA tools like the Ishikawa fish bone diagram, FMEA, and Pareto analysis are used for this purpose [37].

DoE

DoE to optimize NLC has been discussed under the separate heading “DoE in the optimization of NLC”. Through knowledge space, design space is created which is further controlled to give control space (as illustrated in Figure 4) which is a part of continuous improvement after the product is launched in the market [38, 39].

Display full size

Figure 4. 

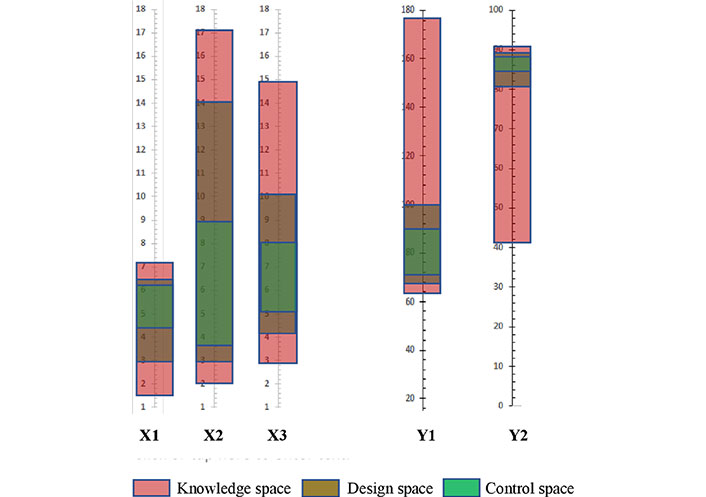
Knowledge space, design space, and control space between input factors (CMAs and CPPs) and CQAs represented on the hypothetical scale. X1: surfactant (%); X2: ST (min); X3: total Lipid (%); Y1: size (nm); Y2: EE (%)

Knowledge space

This is the region of operability during the lab scale product development (Figure 4). After the identification of CMAs and CPPs through screening design, their highest and lowest value is selected. This selection is based upon prior knowledge and experience in formulating NLC where the range of each ingredient and process used is taken into account to develop a stable formulation. This range is called input factors and is used to analyze its relationship with CQAs within the experimental design [39].

Design space

The relationship between input (CMAs and CPPs) and output (CQAs) can be analyzed by design space. Design space can be obtained by the analysis of experimental design. Design space can be of two types. First, if the relationship is analyzed between CMAs and CQAs then this is called product design space and if the relationship is analyzed between CPPs and CQAs then this is called process design space. Within the design space, any change in CMAs and CPPs is permissible independent of the change in the value of CQAs. But if any change is happening outside this design space, then this will impose regulatory restrictions on the formulation and the formulator has to take regulatory approval again for product launch [27].

Control space

From design space, control space is proposed where the range of CMAs and CPPs is further narrowed for future product development. The area of control space within the design space is far from the edge of failure. The purpose of the refinement of CMAs and CPPs is to have further control over CQAs. This is the company’s call to operate in the region of control space as it is not important for regulatory approval [21, 22].

DoE in the optimization of NLC

DoE is a systemic approach to the development of the pharmaceutical product. DoE determines the relationship between input variables, X_i (factors), and outputs, Y (responses) as Y = f(X_i). With fewer experiments, one can find out how changes in factors affect the response [47]. DoE is broadly divided into screening design and response surface design also called optimization design as shown in Figure 5. DoE in optimization experiments generally consists of a selection of appropriate design or design combinations, measurement of response, analysis of response, development of design space, and finally confirmation of design space with at least 3 checkpoint batches.

Display full size

Figure 5. 

Implementation of DoE into pharmaceutical product design

Selection of design

For a large number of factors (factors > 4), one can opt for a screening design before applying an optimization design. However, the screening design can be skipped if the number of factors is less than 4.

Screening design

Risk estimation can be done by either of the most widely used technique in nanoformulation, i.e., FMEA on the basis of RPN scores or screening design.

Plackett-Burman design

Out of different screening designs, the Plackett-Burman design (PBD) is the most widely used for screening risk factors for responses of NLC due to the robustness of this design [48]. PBD is a 2-level factorial design that allows the studying of N number of experiments with N-1 factors. Analysis of Pareto charts (Figure 6) as obtained by running PBD allows picking the most significant factors (ST, concentration of total lipid and surfactant) affecting response (size) while eliminating non-significant factors (stirring speed, stirring time, temperature, and SA) which unnecessarily increases the number of experimental runs. Another example is found in the work of Negi and colleagues [49], where 11 input factors were selected for the optimization of NLC in PBD but just 4 significant factors affecting response were screened and further used for the optimization experiment. PBD is only suitable for factor screening and cannot be used for optimization experiments to find out design space as PBD does not give information on multiple levels of each factor and interaction between factors which also significantly affects responses [32].

Display full size

Figure 6. 

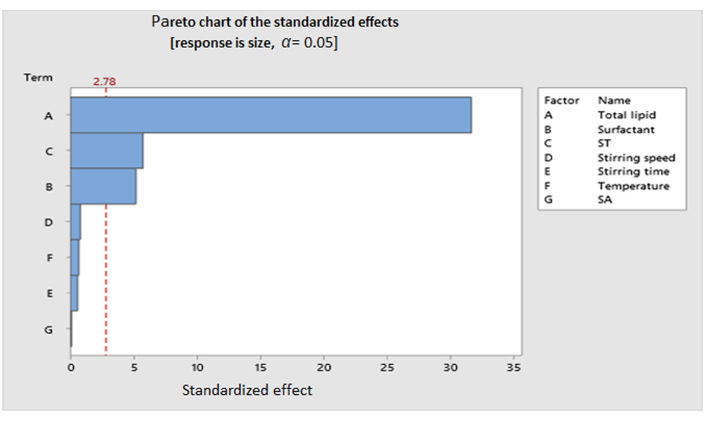
Pareto chart representing the effect of different factors (CMAs and CPPs) on the response (CQAs). Bars crossing the reference line are statistically significant. Bars that represent factors A, B, and C cross the reference line that is at 2.78 (reference line 2.78 was generated by the software Minitab). These factors are statistically significant at the 0.05 level with the current model terms. Factor A is representing the highest effect followed by C and B

Full factorial design

One of the most powerful design to analyze the effect of interaction between input factors on the response is 2-level full factorial design. However, a large number of experiments are required to be performed as compared to other screening designs to screen out significant factors as this design is based on the 2^k model. K represents the number of factors at 2 levels. For 11 input factors, one has to perform 2,048 experiments, unlike PBD which required just 11 experiments to select significant factors [47, 48].

Fractional factorial design

Fractional factorial design (FFD) can also evaluate several factors with a lesser number of experiments like PPD. This is possible if 2-level full factorial design, 2^k is fractionated into 2^{k – p} where p refers to the fraction of FFD, p = 1 for half FFD and p = 2 for quarter FFD. For example, for 6 factors, the number of experiments for 2-level quarter FFD is 2^{6 – 2} = 16 while full factorial design would have given us 2⁶ = 64 experiments. The limitation of FFD is similar to PBD, i.e., interaction terms between factors are not involved thus giving rise to aliased factors [47]. Cacicedo and coworkers [50] studied the effect of multiple factors on responses: size, polydispersity index (PDI), and zeta potential (ZP). Screened factors were further used in central composite design (CCD) for further optimization of NLC [50].

Taguchi orthogonal array

Taguchi orthogonal array (TOA) is an FFD and is represented as L^{k – p} where L, k, and p refer to level, number of factors, and fraction of FFD respectively. For example, for 3 factors and 3 level, L9 TOA give 3^{k – p} = 3^{3 – 1} = 9 experiments to perform. One limitation associated with TOA is that it does not involve the interaction of factors just like PBD and FFD [51]. However, TOA has been used as the main optimization design for NLC in some research publications [49, 52].

Optimization designs

Box-Behnken design (BBD) and CCD are the most commonly used design for the optimization of NLC followed by 3-level full factorial design. Mixture design has also been used in some instances. Studies from the previous three years on formulation optimization of NLC using different experimental designs are represented in Table 4. All these designs are based on a response surface where 3 levels of each factor are used to get a quadratic response surface [47, 53].

Table 4.

Summary of optimization results by the application of QbD on NLC

Experimental design	Drug	CMAs	CPPs	CQA	Results	Reference
4 Factors 3 levels BBD	AmB	mPEG-2K-DSPE, AmB, castor oil	Number of cycles in HPH	PS, PDI, EE, DL	Insignificant effect of all factors on PS and PDI as a quadratic model was not a good fit for these responses which might occur due to aliased factor or human error. Significant –ve interaction between mPEG-2K-DSPE and number of cycles on EE. Increase in concentration of mPEG-2K-DSPE increases EE for 10 cycles but at 30 cycles this effect diminishes. At 0.1% w/v AmB, increase in amount of mPEG-2K-DSPE increases DL, and at 0.3% w/v of AmB, increase in amount of mPEG-2K-DSPE decreases DL as AmB might get saturated with total lipids.	[36]
3 Factors 3 levels BBD	calcipotriol	LL:TL, SC:CSC, calcipotriol	-	PS, EE	Significant effect (+ve) of LL:TL on PS due to aggregation of higher concentration of lipids. Increase in the concentration of SC: CSC decreases PS due to internal arrangement within NLC. Increase in the concentration of LL:TL increases EE due to solubilization of drug inside LL. Increase in the concentration of LL:TL decreases EE as surfactants assisted drug to remain on the surface and inside NLC.	[54]
5 Factors 3 levels BBD + 2 factors 3 levels CCD	Clarithromycin	Lipids, tween 20, PEG 6,000	ST, SA	PS, PDI, ZP, DL, EE	BBD was employed to get the best experimental run with desired CQAs (size in nano, PDI < 0.5, ZP ≥ ± 30 mV, and EE > 80%). CCD was performed using only 2 factors, i.e., CPPs to further improve CQAs. Increased SA significantly decreases EE and LC due to expulsion of drug from NLC. High –ve ZP was observed with increased SA resulting in higher stability of NLC. SA increases temperature of the system resulting in degradation and desorption of non-ionic surfactant which makes –ve value of ZP relatively +ve thus decreasing stability of NLC. PS and PDI decreased with increased SA due to production of shock wave and high temperature which imparts shear force on NLC resulting in breaking of NLC in nano range.	[55]
PBD + 3 factors 3 levels BBD	Ibuprofen	Type of surfactant (P188 and HS15), concentration of surfactant, lipid concentration	Homogenization speed, homogenization time, ultrasonication frequency, ultrasonication time	PS, PDI, ZP, EE	3 CMAs for BBD was screened was ratio of surfactants, concentrations of surfactants and lipid concentration. Quadratic model was found to be significant for size and PDI but insignificant for ZP and EE. Increased lipid and surfactant concentration decreased PS and PDI. Factors were insignificant for ZP and EE.	[56]
Resolution V fractional factorial + 3 factors 3 levels CCD	Metvan	Total lipid, LL, surfactant	ST, SA	PS, PDI, ZP	Total lipid, surfactant, and ST were screened for further optimization. Increased lipid concentration increased PS while decreased PDI. Increased surfactant concentration increased PDI while decreased ZP. PS increased with an increase in surfactant concentration till the middle value, further increase in concentration decreased PS.	[50]
PBD + 3 factors 3 levels CCD	Ibrutinib	LL, drug, surfactant, organic to aqueous ratio	Stirring speed, ST	PS, PDI, EE	LL, drug, and surfactant were screened for CCD as critical factors. Increased amount of drug increased DL resulting in increased surface tension. Thus, increased surfactant concentration was inefficient in decreasing size. Increased PS was observed with increased amount of LL. PDI increased with increased amount of LL and drug. EE increased with increased amount of LL and surfactant.	[57]
23 Full factorial design + CCD	Zidovudine	Tween 80, SL	ST	PS, PDI, DL	Tween 80 and ST were found as critical factors for further use in CCD. As per response surface method, amount of SL:LL and tween 80 was found to be 3:1 and 158 mg.	[58]
3 Factors 3 levels CCD	Efavirenz	Lipid, surfactant	HPH pressure	PS, PDI, EE	PS decreased with increased value of surfactant and HPH pressure while PS increased as lipid concentration increased. On PDI, increased value of surfactant had –ve effect. Moreover, lipid concentration had +ve effect. As HPH pressure increased till intermediate level PDI decreased but after that PDI increased due to increased collision resulting in aggregation of NLC. All 3 factors had significant +ve effect on EE.	[59]
3 Factors 3 levels CCD	Exemestane	SL, surfactant	ST	PS, PDI, EE	PS and PDI decreased with increased concentration of SL till intermediate level. Further increase, increased PS and PDI. Moreover, effect on EE was just reverse of that observed on PS and PDI. PS and PDI decreased with increased surfactant concentrations. PS and PDI increased with increased ST while EE decreased.	[60]
23 Full factorial design	Dexamethasone	Lipid, drug, surfactant	-	PS, ZP, EE	Lipid concentration had no effect on EE. Drug and surfactant concentrations were inversely related to EE and PS. Increased surfactant concentration decreased PS but increased ZP.	[61]
23 Full factorial design	Pterodon pubescens fruit oil	Types of SL, PC, TAS	-	PS, PDI, ZP, TC, EE	TSL has highest –ve impact on PS and PDI. Interaction of TSL × PC and TSL × TAS had significant impact on PS. Lower concentration of P80H, decreased PS. Significant effect of all factors and their interactions (except TSL × TAS) was observed on ZP. ZP value increased by increased amount of TAS and PC while decreased due to increased SL. Highest +ve significant effect of TSL was observed on EE. All other factors were insignificant.	[62]
23 Full factorial design	Zolmitriptan	SL, LL, chitosan	-	PS, PDI, EE, % yield	+ve significant effect was observed with chitosan solution on EE and % yield. EE decreased with increased concentration of SL and LL as drug was hydrophilic but interaction of SL and LL had +ve effect on EE. All factors had significant +ve effect on PS. On PDI SL had –ve effect while LL and chitosan had +ve effect.	[63]
3 Factors 4 levels Taguchi design (L9)	Ergosterol	LL:TL, drug:TL, surfactant, co-surfactant	-	EE	Factors affecting EE was observed in the order: drug:TL > LL:TL > co-surfactant > surfactant.	[52]
5 Factors 2 levels D-optimal mixture design	Hydroxymethyl-nitrofurazone	Gelucire® 50/13, Precirol® ATO 5, Miglyol® 840, P188, water	-	PS	Linear model was significant, R2 = 89.54%. Reduction in PS was observed due to significant interaction observed between gelucire × precirol, gelucire × miglyol, precirol × poloxamer.	[64]

Display full size

D-optimal: determinant-optimal; HPH: high-pressure homogenizer; HS15: hydroxy stearate 15; LL:TL: ratio of LL to total lipids; mV: millivolt; P188: Poloxamer^® 188; P80H: phospholipon 80H; PC: P80H concentration; PEG: polyethylene glycol; SC:CSC: ratio of surfactant concentration to cosurfactant concentration; TAS: types of aqueous surfactant; TC: total content of vouacapans; TSL: type of SLs; -: not applicable; +ve: positive; –ve: negative

Full factorial design

To optimize NLC 2 to 3 factors at 3 levels are often used. The number of experiments is calculated from X^k where X and k refer to the number of level and number of factors respectively. So, for 3 level 3 factors we have to perform 3³ = 27 experiments [47].

CCD

CCD is the most widely used design for optimization. Unlike BBD and full factorial, CCD operates at 5 level for selected 3 level 3 factor experiment. Two levels are –1 and +1 which is factorial design points (Fp), and another 2 levels are –α and +α which is axial points (Ap) and last level are 0 due to center points (Cp). –1, 0, +1 is common to all three response surface design. The value of Ap α is ± 1.682 for rotatable CCD. Number of experiments required for 3 factor 3 level (including two more levels due to Ap) is 2^k + 2k +Cp = 2³ + 2 × 3 + 6 = 20 where Cp is 6 replicas [53].

BBD

3 factor 3 level BBD gives 15 experiments, unlike full factorial design and CCD which requires 27 and 20 experiments respectively. Thus, BBD is economical to perform. This design is also efficient for CCD if we know our safe operating zone. Ap lying outside the cube in CCD are considered outside the knowledge space which is set through lab experiments and is not required to be included as a factor in the experiment [65]. The number of experiments for 3 factor 3 level is 2k (k – 1) + Cp = 2 × 3 (3 – 1) + 3 = 15 [53].

Mixture design

Mixture design is broadly classified as simplex-lattice designs, simplex-centroid designs, and optimal designs. Optimal designs are further categorized as (average) A-optimal, D-optimal, and (integrated) I-optimal [66]. D-optimal is the most commonly used design in optimizing NLC. In mixture design sum of all the components or factors should be equal to 100% or 1 [23]. Suppose, there are 3 components or factors to prepare NLC, i.e., SL, LL, and S_mix with lower and upper ranges as 0.3–0.8, 0.2–0.4, and 0.2–0.4 respectively. The upper range of solid liquid is automatically adjusted to 0.6 to make room for the other two components each with a value 0.2. Therefore, the components will always be under constraint to adjust the sum equal to one. Thus, these components are called dependent factors unlike other optimization designs.

Analysis of response

Significant model and model terms are suggested through analysis of variance (ANOVA). When a model is not significant Box-Cox transformation can provide recommended transformation. Generally, insignificant model terms (P > 0.05) should be removed to improve the model. Lack of fit should always be non-significant [67].

A typical example demonstrating the effect of factors on EE (%) is shown in Figure 7. The factorial plot (Figure 7A) portrays the effect of an individual factor on EE (%). As the concentration of total lipid increases till the intermediate level size increases but after this size decreases. As the homogenization pressure of HPH increases EE (%) decreases due to a negative slope. The same trend is seen with the increase in the number of homogenization cycles but as the slope is less negative the decrease in EE (%) is not that high. The normal plot (Figure 7B) represents factors in red and blue showing the significant and non-significant effects of factors on EE (%) respectively. Factors in red towards the right and the left represents the positive and negative effect on EE (%) respectively. The contour plot (Figure 7C) portrays color coded region. Each colored region indicates how two factors interact to give different values of EE (%) in 2D. Three different regions inside the contour plot have been labeled to show how the interaction between factors changes the value of EE (%). The 3D surface plot (Figure 7D) clearly portrays the effect of factors on response EE (%).

Display full size

Figure 7. 

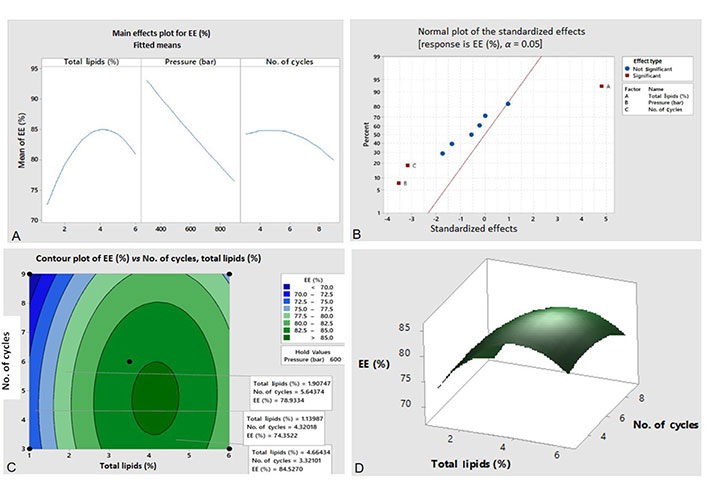
Graphical analysis of EE (%) through (A) factorial plot, (B) normal plot, (C) contour plot, and (D) 3D surface plot

Design space

The optimized formulation can be obtained by desirability function and design space by employing numerical optimization and graphical optimization respectively [68].

Numerical optimization

In numerical optimization, multiple responses are optimized by composite desirability or desirability function. In simple terms, the individual desirability of each response (also called goal) is set and then these individual responses are combined into one called composite desirability [53]. A goal can either be maximizing, minimizing, or targeting responses. In the case of NLC, generally, response like size is minimized while EE (%), LC and percentage in vitro release is maximized [35, 69]. We can also change the value of each factor in numerical optimization. A typical example of numerical optimization of NLC is represented in Table 5 where responses were put under constrain by setting their goals but factors were under no constrain. In numerical optimization, our aim should not be to get composite desirability equal to 1 but to find a set of factors to meet the desired goal as composite desirability depends upon upper and lower limits at which responses or goal is set [53].

Table 5.

Numerical optimization by setting the goal of each response

Response	Goal	Total lipids (%)	Pressure (bar)	No. of cycles	EE (%)	Drug release (%)	Size (nm)	Composite desirability
EE (%)	Maximum	2.86869	463.636	6	86.6053	81.3127	81.5919	0.857581
Drug release (%)	Maximum
Size (nm)	Minimum

Display full size

Graphical optimization

Design space in white color is represented in Figure 8 where critical factors, i.e., total lipid (%) and homogenization pressure (bar) are interacting to give the responses like EE (%), drug release (%), and size (nm) with predefined specifications. The green color region is called knowledge space which is constrained to get design space. Putting further constrain on responses (narrowing down responses) gives control space that lies within the design space.

Display full size

Figure 8. 

Overlay contour plot indicating design space

Conclusion and future perspective

NLC is a widely researched delivery system because of its ability to deliver a wide range of agents like proteins, peptides, hydrophilic, and hydrophobic drugs either through intranasal, oral, ocular, or transdermal routes. NLC is commercially available as a cream and viscous solution at present and there are several patents with different applications. Some researchers have shown the active targeting potential of NLC by surface modification but development in this field is still in its infancy for organ, cellular, and intracellular targeting.

QbD is becoming a widely used technique for formulation optimization inclusive RA across the industry. Implementing define, measure, analyze, improve and control (DMAIC) with six sigma viewpoints in QbD can benefit the commercial production of pharmaceutical products with less likely rejection. The concept of six sigma gives high quality product as there are just 3.4 products per million of the final products that does not meet quality. Six sigma enables production to be 99.9997% perfect [72]. Through the defined approach we identify QTPP, CMAs, CPPs, and CQAs of NLC through risk analysis as given in Tables 1 and 2 respectively. Screening design also helps in identifying CMAs and CPPs. Then the response is measured, which is CQAs, by a using suitable DoE. With the help of statistical process control (SPC), the source of variation and make an attempt to eliminate it. These graphs like control charts give an idea that which experimental run is the source of variation for CQA and then can be controlled further. An alternative to SPC is the use of the ANOVA table as obtained by DoE software where we get an F-ratio. F-ratio automatically gives an idea of whether a model is significant or not. The next step is the analysis of the relationship between factors and responses through model graphs like 3D plots, contour plots, normal plots, factorial plots, etc., as represented in Figure 7. The fourth step is the improvement stage where quality is achieved by DoE and PAT as described in earlier sections. Inside the design space, any change in the factors is permissible as long as CQAs are not lying outside the design space. Here products can be launched into the market. The last step is to have control mainly on quality by continuous improvement in the process. The ranges of the factors are further controlled in order to improve the quality of the products that are already on the market. This control leads to the generation of control space which is not the regulatory requirement as that of working under design space but product quality is beyond the failure range.

Adaptation of QbD in optimizing NLC starts with screening several factors to identify key variables affecting quality characteristics which are further used in optimization experiments to give the best combination of factors to achieve the desired goal. With such advancement, there is still scope for improvement. First, the application of QbD in surface modified NLC with biomarkers, ligands, antibodies, etc. remains to be investigated. Second, the employment of PAT in the production of NLC has not been considered yet which is essential in analyzing and controlling any deviation in critical quality during production itself. QbD is a systematic approach that can be employed in the commercial production of NLC delivering consistent quality without any compromise on safety and efficacy.